
of the kinds of binomial probabilities that you might need to find. at most \(x\), more than \(x\), exactly \(x\), at least \(x\), and fewer than \(x\). We have now taken a look at an example involving all of the possible scenarios. That is, the probability that fewer than 5 people in a random sample of 15 would have no health insurance is 0.8358.

The cumulative binomial probability table tells us that \(P(X\le 4)= 0.8358\). What is the probability that fewer than 5 have no health insurance? That is, the probability that at least one person in a random sample of 15 would have no health insurance is 0.9648. The cumulative binomial probability table tells us that \(P(X\le 0)=0.0352\).

What is the probability that at least 1 has no health insurance? Again, for kicks, since it wouldn't take a lot of work in this case, you might want to verify that you'd get the same answer using the binomial p.m.f. That is, there is about a 25% chance that exactly 3 people in a random sample of 15 would have no health insurance. The cumulative binomial probability table tells us that finding \(P(X\le 3)=0.6482\) and \(P(X\le 2)=0.3980\). What is the probability that exactly 3 have no health insurance? That is, the probability that more than 7 in a random sample of 15 would have no health insurance is 0.0042. The cumulative binomial probability table tells us that \(P(X\le 7)=0.9958\). What is the probability that more than 7 have no health insurance? For kicks, since it wouldn't take a lot of work in this case, you might want to verify that you'd get the same answer using the binomial p.m.f. We've used the cumulative binomial probability table to determine that the probability that at most 1 of the 15 sampled has no health insurance is 0.1671. Let's just take a look at the top of the first page of the table in order to get a feel for how the table works: If you take a look at the table, you'll see that it goes on for five pages.
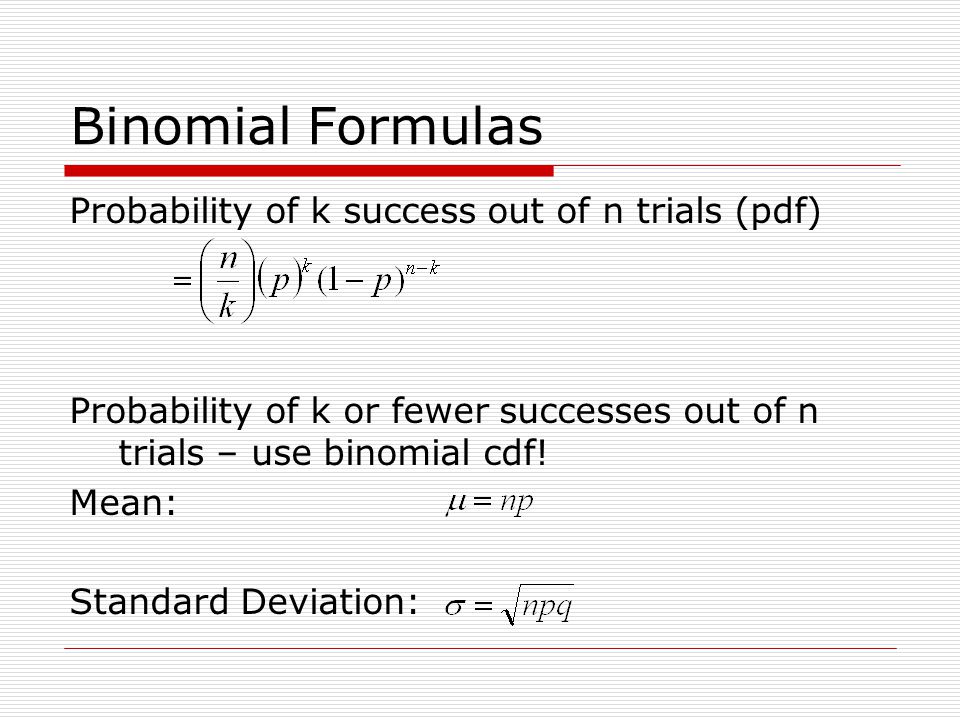
Binomial cdf formula software#
Now the standard procedure is to report probabilities for a particular distribution as cumulative probabilities, whether in statistical software such as Minitab, a TI-80-something calculator, or in a table like Table II in the back of your textbook. You'll first want to note that the probability mass function, \(f(x)\), of a discrete random variable \(X\) is distinguished from the cumulative probability distribution, \(F(x)\), of a discrete random variable \(X\) by the use of a lowercase \(f\) and an uppercase \(F\). For a discrete random variable \(X\), the cumulative probability distribution \(F(x)\) is determined by: Is called a cumulative probability distribution.


 0 kommentar(er)
0 kommentar(er)
